Abstract
We examine the plausibility, scope and risks of aerial transmission of pathogens (including the SARS-CoV-2 virus) through respiratory droplets carried by exhaled e–cigarette aerosol (ECA). Observational and laboratory data suggests considering cigarette smoking and mouth breathing through a mouthpiece as convenient proxies to infer the respiratory mechanics and droplets sizes and their rate of emission that should result from vaping. We model exhaled ECA flow as an intermittent turbulent jet evolving into an unstable puff, estimating for low intensity vaping (practiced by 80-90% of vapers) ECA expirations the emission of 2-230 respiratory submicron droplets per puff a horizontal distance spread of 1-2 meters, with intense vaping possibly carrying hundreds and up to 1000 droplets per puff in the submicron range a distance spread over 2 meters. Bystanders exposed to low intensity expirations from an infectious vaper in indoor spaces (home and restaurant scenarios) face a 1% increase of risk with respect to a “control case” scenario defined by exclusively rest breathing without vaping. This relative added risk becomes 5−17% for high intensity vaping, 40 − 90% and over 200% for speaking or coughing (without vaping). We estimate that disinfectant properties of glycols in ECA are unlikely to act efficiently on pathogens carried by vaping expirations under realistic conditions.
1. Introduction
The current COVID-19 pandemic has brought justified concern and attention to aerial disease contagion through bioaerosols. This contagion is conventionally classified in two modalities determined by the diameter of the aqueous droplets carrying the pathogens, with “direct” exposure associated with large droplets (denoted as “droplets”) that rapidly settle at short distances and “indirect” exposure to small droplets (denoted as “aerosols”) that evaporate before settling, thus remaining buoyant for long periods and spreading large distances, with 5 µm being the conventional cut–off diameter between these two modalities ‡. There is currently a broad consensus, endorsed by the WHO [1] and the CDC [2], that available data supports the occurrence of direct contagion of the SARS-CoV-2 virus by close range exposure to infectious invididuals. While there is also a broad consensus on the factual occurrence of indirect exposure (for example [3]), its scope and relevance still remains controversial [4, 5, 6, 7].
The evolution of bioaerosols spreading disease contagion through respiratory droplets has been widely studied (see [8] and cited references therein). Hence, the current COVID-19 pandemic has motivated the study of direct and indirect aerial transmission of the SARS–COV–2 virus through various expiratory activities, such as breathing, whispering, speaking, singing, coughing and sneezing. The purpose of the present paper is to examine the plausibility, scope and risks of this transmission through a different expiratory route: exhaled e–cigarette aerosol (ECA). While there is currently no factual evidence that pathogens have been spread through this route, it is entirely plausible that this should occur. Since the current COVID-19 pandemic affects millions of vapers and smokers (and non-users surrounding them), we believe that it is an important task to study this transmission route objectively on the basis of available indirect evidence, and to evaluate its involved risks in comparison with other respiratory expirations §.
As opposed to previous studies of aerial pathogen transmission, vaping as a respiratory exhalation would involve a carrier fluid transporting the bioaerosol that is different from air diluted exhaled breath condensate. In this “‘vaporborne” or “ECA-borne” transmission the respiratory droplets would be accompanied by a far larger number of “particles” (droplets) making up the particulate phase of the ECA (bioaerosols particle numbers are far fewer than in non–biological aerosols [8]), with the carrier fluid made of the ECA gas phase diluted in expired air breath. In what follows we provide a quick summary of the section by section content of the paper.
In section 2 we simplify the classification of vaping styles (i.e. the “puffing topographies”) into low intensity ‘mouth to lung’ (MTL) and high intensity ‘direct to lung’ (DTL) styles, with demographic data supporting the overwhelmingly majority status of the former. The physical and chemical properties of ECA are then summarized in section 3 (see extensive reviews [9, 10, 11, 12, 13]).
Since the carried droplets (both the ECA and the respiratory ones) are overwhelmingly in the submicron range, we show in section 4 that ECA is effectively a “single–phase fluid flow” system in which the bulk of droplets become visible tracers of this flow, with the few larger droplets deviating from the flow to follow ballistic trajectories until settling into the ground or being deposited in walls and surfaces.
Given the lack of experimental evidence on respiratory droplets exhaled jointly with the ECA, we need to resort to appropriate respiratory proxies that resemble vaping and on which such evidence exists. To accomplish this task we undertake the following steps: We examine in section 5 the data on respiratory mechanics of cigarette smoking as a proxy to infer and estimate the respiratory parameters of vaping (specially the exhaled volume). This is justified, as most vapers are ex-smokers or current smokers, Since vaping involves mouth inhalation by suction through a mouthpiece, we review in section 6 the available literature on the effects of the inspiration/expiration routes and of mouthpieces and noseclips on respiratory mechanics. (3) Considering the discussion of sections 5 and 6 and looking at available data, we argue in section 7 that mouth breathing can be considered as an appropriate proxy to estimate droplet emission from vaping. The data suggests low emission rates overwhelmingly in the submicron range.
By modeling exhaled ECA flow as a turbulent starting jet with interrupted fluid injection and evolving into an unstable puff, we estimate in section 8 the distance spread for possible direct contagion to be within 1 − 1.5 meters (for MTL style) and 1.5 − 2.5 meters (for DTL style) in the direction of the momentum trusted jet. Once the jet injection (exhalation) terminates the puff is rapidly disrupted by turbulent mixing from entrained surrounding air, with respiratory submicron droplets carried by indoor air flows and remaining buoyant for long times.
To assess the risk of indirect SARS-CoV-2 contagion we consider in section 9 a simplified adaptation to vaping of the exponential dose-response reaction model developed by Buonanno, Morawska and Stabile [14, 15]. We find that the intermittent nature of vaping drastically reduces the added relative contagion risk with respect to the control case of exclusive rest breathing. For a home and restaurant indoor spaces exposure to low intensity vaping just adds about 1% extra risk with respect to the control case scenario. For high intensity vaping this added relative risk is of the order of 5 − 17%, while it rises to 40 − 90% and over 200% if for exposure to vocalizing and coughing (without vaping).
Bactericidal and virucidal properties of glycols contained in ECA, such as PG and VG, have been tested experimentally. However, an examination of the data (section 10) suggests that it is unlikely that environmental disinfection by these glycols could occur under the conditions of normal e–cigarette usage. There is no experimental evidence that disinfection by these glycols would work on the SARS-CoV-2 virus. Nevertheless, appropriate experiments should be set up to probe this possibility even outside the context of vaping.
Finally, in section 11 we provide a detailed summary of results, together with an account of the limitations of the study, its conclusions and policy recommendations. Vaping is an intermittent respiratory activity whose characteristic velocities, droplet diameters and emission rates are comparable to those of breathing and lesser than those of speaking, coughing or sneezing. This implies that in a shared indoor space vaping only adds a minuscule extra contagion risk to risks already existent from rest breathing and other respiratory activities. Setting aside harms from environmental tobacco smoke unrelated to COVID-19, this also applies to sharing an indoor space with a smoker.
2. Vaping styles and demographics
2.1 Puffing topography
Vaping is characterized by a wide range of distinct and individualized usage patterns loosely described by the parameters of puffing topography: puff and inter puff duration, puff volume and flow [16, 17, 18, 19]. This is a factor that complicates the study and evaluation of e–cigarette aerosol (ECA) emissions, more so given the need to upgrade standardization of vaping protocols, specially for the appropriate configuration of vaping machines used for research and regulation. However, in its different topographies some generic characteristics emerge: vaping involves longer puff times and puff (ECA bolus) volumes than conventional cigarette smoking.
To simplify the description of vaping style, we consider two vaping topographies: low intensity “Mouth–To–Lung” (MTL), high intensity “Direct–to–Lung” (DTL), described as follows
TL. It consists of three stages: (1) “puffing”, ECA is sucked orally while breathing through the nose, (2) the puffed ECA is withdrawn from the mouth held in the oropharyngeal cavity without significant exhalation and (3) inhalation into the lungs of the ECA bolus by tidal volume of air from mouth and nose inspiration. It is a low intensity regime involving low powered devices (mostly starting kits, closed systems and recent “pods”) roughly similar to the topography of cigarette smoking.
DTL. As (1) in MTL but bypassing (2): the ECA bolus diluted in tidal volume is inhaled directly into the lung without mouth retention. It is mostly a high intensity regime associated with advanced tank systems.
The topography parameters characterizing these styles are listed in Table 1. It is important to remark that these parameters change when vaping ad libitum in natural environments instead of doing so in a laboratory setting. This was reported in [18]: for example, average puff duration was about 20% longer ad libitum, 5 seconds vs 4 seconds in a laboratory setting.
Puff topography parameters: mb, Vb, Фb are respectively mass (mg) (aerosol yield), volume (mL), flow (ml/sec) per puff of ECA bolus (aerosol yield). Notice that tidal volume VT listed in the table is not the tidal volume for quiet rest breathing (400 − 600 mL), since vaping involves suction of ECA through a mouthpiece (see Sections 5 and 6). Puff time (secs) is tp. Values taken from from rough representative averages from data in figures 1 and 3 of [18] and also from [19].
The table lists various inhaled/exhaled volumes and associated puff times and volumes. The term “puff time” (PT) denotes the time taken to draw smoke from the cigarette (puffng) with “puff volume” (PV) denoting the drawn volume before it mixes with air. Volumes in the second column refer to the inhaled mixture of smoke and air unless it is explicitly specified that it refers to the exhaled mixture. The symbols ±, * and ** respectively denote standard deviation, high and low TAR yields. RIP refers to Respiratory Inductive Plethysmograph, BAT is British American Tobacco.
A third puffing topography not included in Table 1 is “Mouth Puffing”: it shares step (1) of MTL but without step (3), with the ECA bolus diluted in tidal volume air being exhaled without lung inhalation. It is a low intensity regime but involving higher exhaled aerosol density, since less than 5% of aerosol mass is deposited in the mouth [20]. Very few vapers and cigarette smokers use this style, but most smokers of prime cigars and tobacco pipes do.
2.2. Demographics and markets
It is crucial to examine how representative among vapers are the different puff topographies and levels of intensity, something that has varied with time depending on the popularity and availability of different devices. Currently, low powered devices (mostly closed) are the most representative in the largest and most established markets. As shown in figure 1 (Credit to ECig Intelligence [21]) consumer surveys reveal that the overwhelming majority of vapers (80% in the USA) and 90% in the UK) utilize low powered devices (mostly kits for beginners and closed systems), with advanced open tank systems taking the rest.
Usage of different classes of e-cigarette devices in the US and UK markets. Notice that only 15% and 20% of consumers in the USA and the UK use advanced kits that allow for the DTL vaping style (Credit to ECig Intelligence [21]).
The USA and the UK are the biggest and more developed markets, a fact that explains why the closed system category is more prevalent. In a natural evolution of markets the vape category takes off with a more hobbyist segment of users who are more likely to vape with DTL topography in high powered devices that yield large clouds. In nascent markets the ‘easy to use’ open system devices are not of great quality, though recent innovations are likely to improve this. Smokers in large markets are also likely to have higher disposable incomes and a more developed attitude of willing to (and being able to afford to) switch to a less harmful alternative. Such markets also have extensive distribution networks (convenience stores, tobacconists, etc). These factors influence the dominance of the market share of closed system devices and thus to characterize low intensity MTL style as the most prevalent among the vast majority of vapers. Prevalence of mouth puffing (puffing without lung inhalation) is marginal, as an overwhelming majority of vapers inhale to the lung for being ex-smokers or current cigarette smokers.
3. Inhaled and exhaled E-cigarette aerosol (ECA)
The ECA is generated by various physicochemical processes: self–nucleated condensation in a super saturated medium initiates immediately once the e-liquid vapor leaves the coil, the nucleated centers generate small nm scale droplets that grow through coagulation and diffusion [22]. The particulate phase is made of liquid droplets whose chemical composition closely matches that of the e-liquid: propylene glycol (PG), vegetable glycerin or glycerol (VG), nicotine, water [23], together with a negligible contribution of nanometer sized metal particles [24]. The gas phase is chemically similar. The aerosol contains nicotine and residues produced from the pyrolysis of the glycols and the flavorings (mainly carbonyls), which can be in either the gas or particulate phase depending on their vapor pressure and volatility [25], with most of the PG evaporating into the gas phase and VG tending to be remain in the droplets [23].
Count mean diameter (CMD) distributions of mainstream ECA droplets vary depending on the device, puffing style of users, flavors and nicotine content [22, 26]. Droplet number count is heavily dominated by submicron droplets with CMD distributions having either single modes below 100 nm or bimodal forms (one mode well below 100 nm and one in the range 100-300 nm) [22, 26, 27, 28, 29, 30]. However, particle size grows with increasing coil power [26] and even in low powered devices the mass distribution is dominated by droplets larger than 600 nm [22]. In fact, [22] found a third mode around 1 µm that becomes more prominent at increasing power of the tested device while the nm sized modes decrease, likely because higher power involves larger vaporized mass that favors coagulation and scavenging of nm sized droplets by larger droplets.
The inhaled aerosol mass yield depends on the topography parameters given in Table 1. At inhalation of mainstream ECA instrument measured droplet density numbers are in the range n = 1 − 5 × 109/cm3 [26, 27, 28, 29, 30]. Total average droplet numbers of Np = 7.6 × 1010 were reported in [31] for a tank system using e–liquids with high nicotine content in a 2 second machine puff regime ‖ with Vb = 50 mL puff volume (Np decreases 25% with nicotine-free e-liquids). Using the same experimental design [30] reported an increase of up to 30% for 4 second machine puff regime. The estimation Np ∼ 1010 − 1011 is reasonable given a particle number concentration of ∼ 109/cm3 and Vb = 20 − 100 mL of low intensity vaping, with Np ∼ 1012 for high intensity vaping with Vb = 500 mL.
Data on the gas/particle phase partition of the aerosol mass yield mb is roughly: 50% Total Particulate Matter (TPM), 40% PG/VG gas phase, 7% water vapor, < 3% nicotine [32], roughly a similar gas/particulate phase partition to that of tobacco smoke [33]. As shown in [23] and [25] the presence of compounds in gas or PM form depends on their vapor pressure, with PG tending to be gaseous, VG in PM, for nicotine it depends on its PH, while some aldehydes (like formaldehyde) are most likely in the gas phase.
Values of particle numbers and densities for the exhaled ECA can be estimated by considering its retention by the respiratory system. Retention of ∼ 90% of total inhaled aerosol mass was reported in [34] for a wide variety of devices and e–liquids, with the following average compound specific retention percentages: 86% VG, 92% PG, 94% nicotine, while [35] reported 97% total aldehyde retention. This high retention percentages are consistent with the mass distribution of inhaled ECA dominated by larger micron sized droplets which tend to be efficiently deposited in the upper respiratory tracts [22]. Assuming equal retention rate for the particulate and gas phases, we take as total mass of exhaled aerosol and total numbers of exhaled ECA droplet to be 10% of the values of mb listed in Table 1 and 10% of the values of Np = 6.7 × 1010 reported in [31] for a 2 second machine inhalation puff and 50 mL puff volume. Droplet number density of ECA as it is exhaled can be estimated from these values of Np bearing in mind that the exhaled ECA is now diluted in tidal volumes VT listed in Table 1 for the various vaping topographies. This yields number densities in the approximate range np = 106 − 107 cm−3 (lower to higher vaping intensities).
Exhaled ECA dilutes and disperses very fast. Its chemical composition is similar to that of inhaled ECA, both in the gas phase and the droplets [22], with PG and water in the latter evaporating rapidly. Since hyperfine nm sized droplets deposit efficiently by diffusion in the alveolar region and larger micron sized droplets (which tend to grow from hygroscopic coagulation [20, 22]) deposit by impaction in the upper respiratory tracts [20, 31, 26, 28], the CMD distribution of ECA as it is exhaled should be dominated by modes in intermediate ranges 0.1 − 0.5 µm. Since there are no ECA measurements at the exhalation point (the vaper’s mouth), we can estimate the representative droplet diameter by a rough order of magnitude calculation: assuming an aerosol mass yield of 5 mg of inhaled ECA for a low powered device, a 90% retention of aerosol mass with 50% made of PM, the total droplet mass of exhaled ECA should be around Mp = 0.25 mg. Since 90% of droplets are retained, the total number of exhaled droplets should be Np = 7.6 × 109 droplets [31], leading to a median droplet mass of 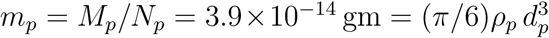 , where ρp is the droplets density that we can assume to be close to VG density: ρp = 1.3 gm/cm3, leading to dp = 0.38 µm. Similar order of magnitud values are obtained for the parameters of high intensity vaping.
, where ρp is the droplets density that we can assume to be close to VG density: ρp = 1.3 gm/cm3, leading to dp = 0.38 µm. Similar order of magnitud values are obtained for the parameters of high intensity vaping.
The fact that CMD chamber measurements are in the range dp = 0.1 − 0.2 µm can be explained by the fact that detectors are located 1-2 meters from the exhalation source, thus measured ECA droplets have already undergone significant degree of dilution and evaporation (as shown in [23] droplets’ mass can decrease by one third in just 1 second by evaporation of its PG content). This is consistent with droplet number densities dropping at least two orders of magnitud from ∼ 106 − 107 cm−3 as they are exhaled to n ∼ 104 − 105 cm−3 at one meter distance from the emission and further dropping to near background levels n ∼ 103 cm−3 at two meters [36, 37, 38].
4. Exhaled ECA as a visualized tracer of respiratory fluid flow
As opposed to other respiratory expirations (breathing, vocalizing, coughing, sneezing), the actual respiratory flow of ECA expiration can be directly visualized. This is a consequence of the optical properties of its particulate phase [8, 39]. Moreover, when visualizing exhaled ECA the viewer is practically seeing nearly the same respiratory air flow that would result from the same respiratory mechanics without involving ECA (i.e. “mock vaping”). This is so because of its physical properties: exhaled ECA is a “single–phase fluid flow” (SFF) system [40, 41] in which the particulate phase (made of overwhelmingly submicron ECA droplets) have negligible influence on the fluid dynamics, acting essentially as visible tracers or (to a good approximation) as molecular contaminants carried by the fluid.
The fact that exhaled ECA can be used as an effective tracer of respiratory flows is not surprising nor unique, given the existence of numerous gas markers and aerosols in a SFF regime that are widely used as proxies for the study and visualization of expired air [42, 43]. This also applies to mainstream exhaled tobacco smoke, whose particulate matter is also made of submicron liquid and solid droplets. In fact, there are studies that have directly used cigarette smoke as a tracer to visualize respiratory airflows [44, 45, 46]. It is worth mentioning that respiratory droplets potentially carried by exhaled ECA would not change its possible role as a tracer of expiratory flows, since as we show further ahead (section 7) these droplets are also overwhelmingly in the submicron range and their numbers are much fewer than ECA droplets.
The particulate phase of the ECA at exhalation consists of submicron droplets made of PG, VG, water and nicotine, whose density should be close to VG density ρp ∼ 1.3 gm/cm3. Dilution and PG evaporation further reduce droplets diameters and number densities. These droplets are evolving along a carrier fluid made of a gas mixture: the gas phase of the ECA strongly diluted in exhaled air (in practice, we can think of the carrier fluid simply as exhaled air at mouth temperature ∼ 30 − 35° C). As in all SFF systems, In what follows we examine two criteria that determine the SFF character of the exhaled ECA: the particles’ relaxation time and volume fraction.
Submicron particles in a carrier fluid have little inertia, and thus essentially follow the fluid flow. They are well within the Stokes regime with Reynolds numbers Rep ≪ 1 and negligibly small relaxation times trel, the response time of an aerosol particle to adjust to external forces. For dp = 0.3 µm we get [39]
 where µ = 1.895 × 10−5gm/(sec cm) the dynamic viscosity of air at 35 C and Cc = 1 + (λ/dp)[2.34 + 1.05 exp(−0.39dp/λ)] ≈ 1.4 is the Cunningham slip factor with λ = 0.066 µm the mean molecular free path of air. The relaxation time provides the time scale for a particle released into a fluid with velocity U along a horizontal stream to settle into the fluid velocity (neglecting gravity). In this case (see Chapter 3 of [39]) the velocity of the particle
where µ = 1.895 × 10−5gm/(sec cm) the dynamic viscosity of air at 35 C and Cc = 1 + (λ/dp)[2.34 + 1.05 exp(−0.39dp/λ)] ≈ 1.4 is the Cunningham slip factor with λ = 0.066 µm the mean molecular free path of air. The relaxation time provides the time scale for a particle released into a fluid with velocity U along a horizontal stream to settle into the fluid velocity (neglecting gravity). In this case (see Chapter 3 of [39]) the velocity of the particle 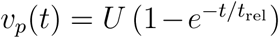 becomes practically identical to U in about 10−5 seconds (instantaneously in practical terms), thus justifying the notion of particles simply following the fluid flow with (practically) no influence on its dynamics. This behavior occurs also for the larger ECA droplets of dp ∼ 1 µm whose relaxation times are
becomes practically identical to U in about 10−5 seconds (instantaneously in practical terms), thus justifying the notion of particles simply following the fluid flow with (practically) no influence on its dynamics. This behavior occurs also for the larger ECA droplets of dp ∼ 1 µm whose relaxation times are 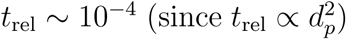 . Evidently, these relaxation times are much smaller than macroscopic characteristic times of the carrier fluid (for example a 2 second inhalation time or even the tenths of a second the ECA stays in the mouth cavity [20]). The Stokes number is defined as St = trel/tf, where tf is a characteristic fluid time, hence for the xhaled ECA we have St ≪ 1, which is another criterion to define SFF systems. Another criterion for an aerosol to be described as SFF systems is the ratio φ of total volume of the particles to the fluid volume satisfying [40, 41]
. Evidently, these relaxation times are much smaller than macroscopic characteristic times of the carrier fluid (for example a 2 second inhalation time or even the tenths of a second the ECA stays in the mouth cavity [20]). The Stokes number is defined as St = trel/tf, where tf is a characteristic fluid time, hence for the xhaled ECA we have St ≪ 1, which is another criterion to define SFF systems. Another criterion for an aerosol to be described as SFF systems is the ratio φ of total volume of the particles to the fluid volume satisfying [40, 41]
 where Np is the total number of particles,
where Np is the total number of particles, 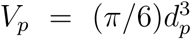 is the particles’ volume (assuming they are spherical) and Vf is the fluid volume. Substituting the qualitative values we obtained for the exhaled ECA: Np = 7.6 ×109, dp = 0.3 µm and an exhaled air volume of Vf = VT = 300 − 1500 mL for a low intensity regime yields φ = 1 − 3 × 10−7, which fulfills (2). This condition holds even if we assume that a large part of the mass distribution is contained in micron sized (dp ∼ 1 µm) droplets making (say) 10% of the total number. The value of φ is bound to decrease as the exhaled ECA dilutes and the volatile droplet compounds (PG and water) evaporate. As shown in [23] this process decreases the droplets mass (and thus volume) to one third while the fluid volume increases and thus φ necessarily decreases.
is the particles’ volume (assuming they are spherical) and Vf is the fluid volume. Substituting the qualitative values we obtained for the exhaled ECA: Np = 7.6 ×109, dp = 0.3 µm and an exhaled air volume of Vf = VT = 300 − 1500 mL for a low intensity regime yields φ = 1 − 3 × 10−7, which fulfills (2). This condition holds even if we assume that a large part of the mass distribution is contained in micron sized (dp ∼ 1 µm) droplets making (say) 10% of the total number. The value of φ is bound to decrease as the exhaled ECA dilutes and the volatile droplet compounds (PG and water) evaporate. As shown in [23] this process decreases the droplets mass (and thus volume) to one third while the fluid volume increases and thus φ necessarily decreases.
Evidently, larger droplets (diameters larger than a few µm) are present in ECA particle diameter distributions and such particles should contain a significant portion of the aerosol mass [22], but they are too few in numbers and deviate from the flow following ballistic trajectories, thus do not affect the dynamics of the carrier fluid to consider ECA as a biphasic fluid flow system.
5. Vaping as a respiratory process
Since most vapers are either cigarette smokers or ex–smokers of cigarettes, it makes sense to examine the respiratory parameters of smoking as reported in the literature (see reviews in [47, 48], see also Table 3). Tobacco smoke is a valid reference for ECA, as it is also an aerosol in a SFF regime characterized by a particulate phase made of predominantly submicron particles with similar particle numbers and diameter distributions [22, 49, 50] (though the particulate and the gas phases of each aerosol have very different chemical properties).
The symbols Np and np stand for droplet number per exhalation and average droplet number density (cm−3). LE, HE, BH, L, and exh are Low emitters, High Emitters, Breath Hold, litter and exhalation. The acronyms OPC, EM, APS, SMPS, CNC stand for Optical Particle Counter, Electron Microscopy, Aerodynamic Particle Sizer, Scanning Mobility Particle Sizer, Condensation Nucleus Counter. The subjects in all studies (save possibly [83]) breathed through a mouthpiece wearing a noseclip.
While there is a wide individual diversity in respiratory parameters among smokers, roughly three patterns of smoking topography can be identified [51] that are analogous to the vaping topographies examined in Section 2: MTL, DTL regimes and Mouth Puffing (with tobacco smoke instead of ECA). As with vaping, the most common cigarette smoking topography is MTL, an expected outcome since most vapers are either ex-smokers or current smokers of cigarettes. While a sizable minority of 10-20% of vapers (see Section 2.2) follow the DLT pattern, the vast majority of smokers avoid direct lung inhalation because it is too irritant (and is consistently associated with airways narrowing [51]). Among 10 smokers examined by Tobin et al [52] only one inhaled directly to the lungs as revealed by expansion of the abdomen at the same time as puffing. In fact, preference for the “normal” MTL pattern is very likely an organic response to minimize to a tolerable level the irritant quality of tobacco smoke [51, 52, 53]. Also, it is likely that the demographic preference for the MTL style among vapers follows from the fact that most are either recent ex-smokers or current cigarette smokers who simply vape following a familiar usage pattern.
Few cigarette smokers and vapers follow a Mouth Puffing topography, but the latter is the preferred pattern among most cigar and tobacco pipe smokers. The physiological differences between Mouth Puffing and MTL patterns was examined by Rodenstein and Stanescu in an observational study [54] involving 43 subjects: 6 primary and 6 secondary smokers of tobacco pipe, 20 cigarette smokers and 11 never smokers. They found that in all pipe smokers (save one) oral smoke inhalation and breathing only with the nose remained separate processes taking place with the oropharyngeal isthmus closed (see further discussion on this in Section 6) to prevent overt lung inhalation of smoke. However, the two processes subsequently interfered with each other once the soft palate and tongue separate to open the oropharyngeal isthmus to allow a deep lung inhalation of the retained smoke bolus in the oropharynx by joint mouth and nose breath inspiration. As a consequence of these differences, most pipe smokers keep a fairly regular breathing pattern similar to that of normal rest breathing with small fluctuations of tidal volume, while cigarette smoking is characterized by large and irregular tidal volume fluctuations markedly distinct from normal breathing.
Regarding its respiratory parameters, cigarette smoking involves 20-25% of the vital capacity [47], though low intensity inhalators might use on average only 14% [53]. Other parameters such as tidal volume, puff times and volumes obtained in observational studies are listed in Table 2, where we used outcomes from references cited in two comprehensive reviews [47, 48]. These outcomes are roughly:
Puff Volume” (volume of the smoke bolus drawn from the cigarette) 20-70 mL,
Puffing Times (time to draw the smoke bolus from the cigarette) ∼ 2 seconds
Total smoking time lapses (inhalation, breath hold and exhalation) ∼ 4 seconds
Tidal volumes (the volume of the total inhaled/exhaled smoke mixed with air, VT in table 1) vary widely between 300 and 1500 mL (with some outliers reaching close to 2000 mL), but typically group averages are between 700 and 900 mL
It is worth remarking that puffing times are slightly shorter but roughly comparable to those of MTL vapers, while tidal volumes are 25-30% larger than rest tidal volumes (400-600 mL), though the measurement of these volumes is subject to at least a 10% error [55] and also, not all air drawn with the purpose of inhaling smoke is actually inhaled. Most studies report inhaled volumes, but exhalation volumes are roughly comparable (see Table 2), as smoke is highly diluted in air and its retention barely affects volume measurement.
As opposed to rest breathing, smoking and vaping involve suction: the inward force needed to draw smoke (or ECA) associated with the negative/positive pressure gradient ΔP generated by the diaphragm driven expansion/contraction of the lungs. Airflow resistance follows from the relation between the flow of air volume Q = dV/dt and this pressure gradient, a relation that can be modeled by the power law [60, 61]
 where a, b are determined empirically. This power law can be related to fluid dynamics (see discussion in [61]): the constants a and b correlate with fluid density, while the exponents b can be referred to the “classical” flow regimes: b = 1 corresponds to laminar flow with Reynolds numbers Re < 10 (Pouseuille law), b = 1.75 to turbulent flow Re ∼ 10000 (Blasius law) and b = 2 is the “orifice” flow characterized by turbulent flow in narrow pipes and containers.
where a, b are determined empirically. This power law can be related to fluid dynamics (see discussion in [61]): the constants a and b correlate with fluid density, while the exponents b can be referred to the “classical” flow regimes: b = 1 corresponds to laminar flow with Reynolds numbers Re < 10 (Pouseuille law), b = 1.75 to turbulent flow Re ∼ 10000 (Blasius law) and b = 2 is the “orifice” flow characterized by turbulent flow in narrow pipes and containers.
The theoretical connection with fluid mechanics has motivated airflow resistance measurements in the upper respiratory system that yield values around b = 1.84 [60, 61] for resting oral and nasal breathing. An excellent fit of this power law relation to the classical orifice flow b = 2 was found for a conventional cigarette and a two second generation e–cigarettes [50], with the e–cigarettes flow resistance a between 3-4 times larger than the conventional cigarette. As a consequence, given the same suction effort (same ΔP) a conventional cigarette yields a puffing flow 𝒬 between 3-4 times larger than the tested e–cigarettes (second generation). However, vapers can compensate the higher flow resistance of ECA and draw relatively large aerosol mass with the same suction effort by puffing for longer times (as shown by topography studies). Also, the laboratory measurements in [50] were conducted under idealized conditions and are very likely to vary among the many e-cigarette devices in natural usage conditions.
A factor that distinguishes cigarette smoking from vaping is that the latter involves suction of ECA through a mouthpiece. However, in most of the studies listed in Table 2 the subjects smoked through cigarette holders that are part of the laboratory instrumentation. This makes the listed outcomes more useful to infer respiratory parameters for vapers, at least for those vaping in the MTL style, since these holders are of similar size and shape as the narrow e–cigarette mouthpieces. Though, usage of cigarette holders does not seem to introduce significant changes in tidal volume, as can be seen by comparing outcomes from studies that used holders with those who did not in Table 2 (we comment further on the effect of mouthpieces in Section 6).
Since MTL is the most common topography among smokers and vapers (most of whom are ex-smokers or current smokers), we can assume that MTL style vaping is characterized by qualitatively similar puffing and respiratory parameters to those listed in Table 2. While some smokers inhale without a mouth hold as in DTL style, this does not seem to involve in them a significantly higher tidal volume, most likely because it can be too irritant [51, 53]. The lesser irritant nature of ECA is a plausible explanation for a larger proportion of vapers that can tolerate DTL topography, which means suction of a much larger aerosol mass [19, 62] and thus significantly larger puffing and tidal volumes than in MTL style (made easier by usage of high powered devices). A puff volume of 500 mL can yield under idealized laboratory conditions an inhalation tidal volume close to 3 LT [63], which justifies the more plausible values listed in Table 1.
6. Effects of mouthpieces and noseclips
Mouthpieces (MP) and nose-clips (NC) (to block nasal inspiration) are standard instruments in observational studies, not only those aimed at studying droplet emission, but of respiratory patterns and flows in human subjects. Since the results of these studies can serve as appropriate proxy values to infer droplet emission in vaping, it is important to assess the effects of these instruments in respiratory mechanics. For the purpose of the present article, this issue is interesting because ECA is inhaled in e–cigarettes through mouthpieces (though without obstruction of nasal breathing).
Several studies conducted in the 1970’s and 1980’s [64, 65, 66, 67, 68] have shown that breathing through MP’s and NC’ affect all respiratory parameters with respect to unencumbered nose breathing: while tidal volume increases roughly 20% with respect to its normal rest value of 400-600 mL in all studies, inhalation and exhalation times and respiratory frequency are much less affected. In [68] a NC without a MP produces a similar increase of tidal volume but also significant increase of inhalation times (15%) and exhalation times (22%). Two of the studies [64, 65, 68] were conducted on subjects in supine position, but different body positions only produce minor variation of respiratory parameters [69].
Besides possible reasons like the psychological sensorial stimulation of receptors by colder air in mouth inspiration and the stress of breathing through instruments, another possible explanation for the observed change in respiratory parameters of MP’s is the change of airflow resistance, for example: a 70–90% reduction [68] brought by the large added mouthpiece dead space (up to 80 mL), while the larger airflow resistance from the standard 17 mm to a narrower 9 mm MP (closer in size to mouthpieces used in vaping) reduced the increase of tidal volume to 11% and inhalation/exhalation times to 9% [68]. Therefore, the MP’s of e-cigarettes should not produce significant modifications of respiratory parameters.
The relation between airflow resistance and MP diameter follows from comparing fluid flow in the MP with that along a Venturi-meter tube in which the Reynolds number is Re = 4ρ 𝒬/(π µ d), with ρ, µ the fluid density and dynamical viscosity and d the tube diameter. The pressure gradient vs flow 𝒬 is given by (3), which for the expected turbulent flow in a MP (negligible effect of µ) can be expressed in terms of ρ and d (the MP diameter) qualitatively as ΔP ∝ (ρ𝒬2)/d4 (see [61]). Hence, in a comparison of two e–cigarette MP’s the same suction effort (ΔP)2 = (ΔP)1 yields for the MP with larger diameter (d2 > d1) a larger flow 𝒬2/𝒬1 ∝ (d2/d1)4.
In the studies discussed above there was no separation between usage of instruments (MP & NC) and oral breathing. Rodenstein, Mercenier and Stanescu [70] conducted several experiments with 14 healthy subjects with the aim of looking separately at the effects of MP’s and a NC’s. Their results show that breathing through a MP without a NC (with and without instructing the subjects on how to breath) practically keeps all respiratory parameters identical to those of normal nasal breathing with closed mouth: resting tidal volume barely changed from 533 ± 253 to 559 ± 284 mL, breathing cycle (time for inspiration and expiration) practically remained the same at 4.8 ± 2.3 and 4.9 ± 1.8 seconds. They observed that 9 of 14 subjects breathed in a normal manner even if their mouth was connected to a MP. However, they observed qualitatively the same changes as [64, 65, 66, 67, 68] with subjects breathing through an MP plus NC: tidal volume increased to 699 ± 415 mL and inhalation/exhalation time to 5.5 seconds.
The main result of Rodenstein et al is that changes of respiratory parameters (rough 20% and 10% increase of tidal volume and inhalation/exhalation cycle) are entirely due to the forced oral breathing induced by the NC, in fact, nose occlusion is not even necessary to produce these changes: it is sufficient to simply instruct the subjects to breath through the mouth to observe an increase the tidal volume by a similar proportion as with the use of a NC: from 456 ± 142 to 571 ± 199 mL, though inhalation/exhalation times and other parameters remain almost the same (likely because of breathing without instrumentation).
The physiology behind the effects of the breathing route is similar to the one discussed in the study of pipe and cigarette smokers [54]: changes of respiratory parameters depend on the degree with which subjects are able to maintain air flowing through the nose. These parameters exhibit minor variation as long as this air flow is not occluded and the oropharyngeal isthmus remains closed. The parameters change significantly when nose occlusion separates the soft palate and the tongue and opens the oropharyngeal isthmus to allow air to flow entirely through the mouth. However, after the initial puffing, air flows through both nose and mouth in smoking and vaping (except the Mouth Puffing style), with the soft palate closing and rising enough to control the oral or nasal flow.
7. Diameter distribution and rate of emission of respiratory droplets potentially carried by exhaled ECA
The discussion in the previous sections has allowed us to infer the characteristics and parameters of the respiratory mechanics of vaping. We need now to identify among respiratory processes the ones that most closely fit these parameters in order to use their available experimental data to infer the capacity of vaping for respiratory droplets emission.
7.1. The right respiratory proxy: mouth breathing
Given the fact that exhaled ECA is a single phase flow (SFF) system (see section 4), a good criterion to relate vaping to other respiratory processes is the comparison between its fluid exhalation velocity U0 and measured analogous velocities in other respiratory processes.
The exhalation velocity U0 can be roughly inferred qualitatively by considering an exhaled tidal volume of fluid flowing through the respiratory tracts. Considering the respiratory parameters discussed in the previous sections (summarized in Table 1) we can use the simple approximate formula
 where VT is the exhalation tidal volume (in cm3), texh is the exhalation time in seconds and A is the combined mouth and nose area (in cm2), as the fluid carrier of both ECA and tobacco smoke is exhaled through the mouth and nose. From the values listed in Tables 1 and 2 we have:
where VT is the exhalation tidal volume (in cm3), texh is the exhalation time in seconds and A is the combined mouth and nose area (in cm2), as the fluid carrier of both ECA and tobacco smoke is exhaled through the mouth and nose. From the values listed in Tables 1 and 2 we have:
MTL vaping and smoking: VT = 300 − 1500 mL and texh = 2 − 3 sec., while values for the combined mouth/nose area has been measured between A = 2 − 3 cm2 [45].
DTL Vaping: VT = 1000 − 3000 mL with texh ≈ 3 − 4 sec. and A ≈ 3 cm2. Given the large amount of exhaled fluid we assume longer exhalation times and larger mouth opening area.
From the combination of the parameter values mentioned above we have
 which indicates that mouth breathing is the appropriate respiratory proxy for MTL vaping and cigarette smoking, as well as the less intense DTL regime (up to 300 cm/sec), since these estimated exhalation velocities are well within the range of those of exhaled breath in mouth breathing without nose occlusion by NC’s [71, 72, 73], which have been estimated and measured by various techniques (including Schlieren photography). Exhalation velocities in the most intense DTL vaping regime approach in their upper end the velocities of vocalizing but fall short of those of coughing and sneezing. As a reference, measurements of U0 using Particle Image Velocimetry resulted in averages of 3.9 m/s for speaking and 11.7 m/s for coughing [74] (measurements in [75] resulted in 6-22 m/s with average 11.2 m/s for coughing), while 35 m/s has been estimated for sneezing [76, 77, 78].
which indicates that mouth breathing is the appropriate respiratory proxy for MTL vaping and cigarette smoking, as well as the less intense DTL regime (up to 300 cm/sec), since these estimated exhalation velocities are well within the range of those of exhaled breath in mouth breathing without nose occlusion by NC’s [71, 72, 73], which have been estimated and measured by various techniques (including Schlieren photography). Exhalation velocities in the most intense DTL vaping regime approach in their upper end the velocities of vocalizing but fall short of those of coughing and sneezing. As a reference, measurements of U0 using Particle Image Velocimetry resulted in averages of 3.9 m/s for speaking and 11.7 m/s for coughing [74] (measurements in [75] resulted in 6-22 m/s with average 11.2 m/s for coughing), while 35 m/s has been estimated for sneezing [76, 77, 78].
7.2. Droplet emission from mouth breathing
There is an extensive literature on respiratory droplets emitted by mouth breathing at different levels of lung capacity, including rest tidal volume breathing (< 20% of vital capacity). We list a selection of the latter studies in Table 3, as they are the ones that can serve as proxies for vaping and smoking (at least MTL style). In practically all the listed studies subjects breathed through MP’s (mouthpieces) and NC’s (noseclips), which as discussed in section 6, involves occlusion of nasal air flow that implies a slightly modified mechanics and about 20% larger tidal volume with respect to normal unencumbered breathing.
While some of the studies in Table 3 were motivated by investigating droplet emission in the context of airborne pathogen contagion [79, 80, 81, 82], the motivation of others [83, 84, 85, 86, 87, 88] is to probe various mechanisms of droplet formation (see comprehensive discussion and reviews in [77, 89, 90]), specifically the airway reopening hypothesis of small peripheral airways that normally close following a deep expiration, which was further tested by computerized modeling [90] that simulated this mechanism of particle formation by rupture of surfactant films involving surface tension. The mechanism was probed in [83] by showing that concentrations of exhaled particles significantly increase with breathing intensities higher than rest tidal volume, but also for fast exhalations but not fast inhalation, while droplet numbers increased up to two orders of magnitude: from ∼ 230/Lt in tidal volume (0.7 Lt) to over 1200/Lt in a breathing maneuver from fractional residual capacity to total lung capacity [85].
The difference in droplet formation between breathing and speaking was examined in [91]: normal and deep tidal breathing produced submicron distributions related to those of other studies probing the airway reopening mechanism, while speech and cough produced larger diameter modes (∼ 1µm) with particle formation associated with vocal cord vibrations and aerosolization in the laryngeal region. A third mode of median diameters of 200µm was associated with the presence of saliva between the epiglottis and the lips.
Breath holding between inspiration and expiration were found in [83] to significantly reduce concentrations of exhaled droplets in proportion to the breath hold time. The same outcome resulted in [92] for inspiration to total lung capacity, but droplet numbers increased when the breath hold occurs before inspiration. These outcomes fit predicted effects of gravitational settling in the alveolar region. Since observations in [83, 92] involved breathing intensity well above tidal volume up to total vital capacity, it is not possible to compare them quantitatively with the breath hold of the MTL style. However, gravitational settling of larger droplets must also occur in the bucal cavity under normal vaping conditions [20], so it is reasonable to assume that reduction of exhaled droplet numbers should also occur at lower intensity in MTL style vaping. The fact that emitted respiratory droplets in tidal breathing are overwhelmingly in the submicron range implies a very rapid evaporation (0.01 sec) that in practice can be considered as instantaneous, with the emitted disiccated droplets (droplet nuclei) made of salt crystals and lypoproteins and being about roughly half [93] their original diameter. The exhaled breath will also contain some larger particles dp ∼ 1 − 3 µm that evaporate in timescales of 0.1 sec. As a consequence, relative humidity bears negligible influence on the droplets evolution.
7.3. The resulting picture
It is important to pause and gather all the evidence and data from this and previous sections. It is plausible to assume that droplet emission in smoking and vaping (at least MTL style) can be reasonably inferred from outcomes of studies in Table 3 with comparable exhaled tidal volumes (see Table 2), including outcomes of studies mentioned previously that examined breath holds.
The studies we have summarized and listed in Table 3 only involve mouth breathing, but share some common respiratory features with vaping and smoking: oral inspiration with usage of MP’s (in vaping), as well as qualitatively similar exhalation velocities and respiratory parameters: inhalation/exhalation times and tidal volumes. However, there are also differences: smoking and vaping do not involve the nose occlusion of these experiments, but involve suction which the subjects of the latter experiments did not experience. While absence of NC’s would imply a tidal volume very close to rest values in MTL smoking and vaping, this absence is compensated by the increase due to the need to overcome airflow resistance through suction. The decrease of droplet emission from the mouth/oropharynx hold in MTL topography (absent in normal breathing) was a detected outcome in two of the studies listed in Table 3. We have then the following inferences regarding emission of respiratory droplets
MTL vaping and smoking (and even DTL vaping not involving deep inspiration). The outcomes displayed in Table 3 suggest that exhaled droplets should be overwhelmingly in the submicron range (typically peaking at dp = 0.3 − 0.8 µm) and a small rate of droplet emission: roughly Np = 2 − 230 per exhalation (per litter), with droplet number densities well below np = 1 cm−3, though the wide individual variation reported in these studies should also apply to vaping, including the existence of a small minority of individuals that can be thought of as “super emitters” reaching over Np ∼ 1000 per exhalation.
DTL vaping. It involves a spectrum of deeper respiratory intensity than MTL vaping and thus should involve a higher rate of droplet emission. Perhaps the closest analogue in the studies listed in Table 3 to infer droplet emission for intense DTL (2-3 LT exhalation) breathing at fractional residual capacity in [85] that reported emission rates of around 1000/LT. However, this style of vaping is practiced by a small non-representative minority of vapers (roughly 10-15%, see figure 1), while extreme vaping with big clouds (the so called “cloud chasers”) is even less frequently practiced in competitions or exhibitions. Evidently, this type of vaping cannot be sustained for long periods.
8. Airflow dynamics
In the previous sections we have inferred the submicron characteristics and rate of emission of respiratory droplets expected to be carried by exhaled ECA. We need to estimate now how far can these respiratory droplets be carried to evaluate the distance for direct exposure of bystanders to pathogens potentially carried by these droplets
Exhaled ECA is injected into surrounding air a given horizontal distance roughly in the direction of the exhaled flow. Since it involes a finite fluid mass of a SFF aerosol during a finite injection time (exhalation time), the appropriate dynamical model for it is a turbulent puff with a starting momentum dominated jet that lasts while the fluid injection is on [94, 95, 96, 97, 98, 99, 100, 101]. A schematic description of this system is furnished by Figure 4. We will not be concerned with the few large particles (diameters d ∼ 3 − 5µm and over) that initially follow the fluid stream but (depending on their size) exit the main flow to follow ballistic trajectories until they either deposit on surfaces, settle on the ground or evaporate [77, 102].
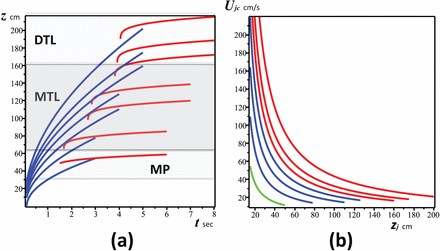
Puff and initial Jet with axial symmetry. The stating jet is propelled by linear momentum parallel to the centerline velocity Uc, the arrows above and below represent the entrainment velocity mixing surrounding air with the carrier fluid. As the uid injection terminates (end of exhalation), the entrained air makes about 40% of the fluid mass making the the transition into a ellipsoidal puff through highly turbulent vortex structures. At this point the puff is likely to disperse rapidly as horizontal displacement velocities are comparable to velocity uctuations characterizing high turbulence and thermal buoyancy.
Jet/Puff horizontal displacement and centerline velocity. Panel (a) displays the displacement zjc of the staring jet (blue) and zpc of the puff (red) as functions of time from (equations (7) – (10)), for the three vaping topographies described in section 2: DTL (Direct to Lung), MTL (Mouth to Lung) and MP (Mouth Puffing). We assumed as injection (exhalation times) 3, 4 and 5 seconds. The initial velocities from top to bottom are U0 = 50; 100; 150; 200; 250; 300; 400 cm/s. Panel (b) depicts centerline velocities Uc for the starting jet (equation (8)), as functions of the horizontal displacement zjc during the injection times and initial velocities of panel (a) (green for MP, blue for MTL and red for DTL). Notice that once injection stops the jet has reached velocities comparable to those of indoor air currents.
The curves display ERq (quanta/hour) as a function of viral load cv (RNA copies/mL) for various expiratory activities: rest breathing (br), low and high intensity vaping (vp), speaking (bottom to top) 10; 20; 30; 40% of the hour (sp), coughing (cf) and speaking 100% of the time (sp100). Numerical values of ERq for cv = 107 RNA copies/mL (vertical line) are listed and discussed in the text. The ratios between these activities and rest breathing (taken as the case control scenario) is displayed in figure 7.
Given the distance and time dispersion scales (< 3 meters and < 2-3 minutes) we can approximate the ECA as an airflow at constant atmospheric pressure, air density and dynamical viscosity ρa and µ. For a jet source (vaper’s mouth) approximated as an orifice of 1.5 − 3 cm2 area [45] (diameter d0 =1.25-1.75 cm) and initial velocities U0 given by (5), exhalation Reynolds numbers Re = (ρ/µ)U0d0 = 600 − 4400 are in the transition between laminar and turbulent, values well below the high Reynolds numbers expected near a jet source [94, 95], but we are mostly concerned with the jet evolution and displacement (penetration) along horizontal distances z »d0. Other parameters to consider are the injection time texh = 2 − 5 seconds and a temperature gradient from exhalation (initial) T = 30° − 35° C (mouth temperature) into an assumed T = 20° C for the surrounding air. For such values and scales the starting jet can be regarded as isothermal with thermal buoyancy becoming relevant only in the puff stage [98, 99].
It is well known that steady and unsteady jet/puff systems can be well approximated by analytic models that assume axial symmetry and a self similar profile for the average centerline and radial components of the velocity field in cylindrical coordinates  [94, 95, 103, 104] (see figure 4)
[94, 95, 103, 104] (see figure 4)
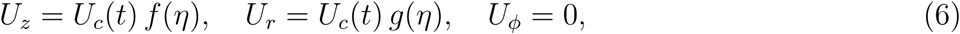 where f, g are empiric Gaussian or polynomial functions of the self similar variable η = r/z and the centerline velocity is Uc = Uz for r = 0 along the z axis, hence f (η), g(η) must satisfy Uz = Uc and Ur = 0 at r = 0 (see examples in [77, 94, 95, 96, 97, 98, 99, 100, 101]). An axially symmetric self similar jet/puff system fulfills the conservation of linear specific momentum Q = V Uc (puff) and force
where f, g are empiric Gaussian or polynomial functions of the self similar variable η = r/z and the centerline velocity is Uc = Uz for r = 0 along the z axis, hence f (η), g(η) must satisfy Uz = Uc and Ur = 0 at r = 0 (see examples in [77, 94, 95, 96, 97, 98, 99, 100, 101]). An axially symmetric self similar jet/puff system fulfills the conservation of linear specific momentum Q = V Uc (puff) and force 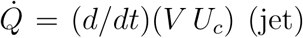 where V is the penetration volume [94, 100, 101], hence
where V is the penetration volume [94, 100, 101], hence 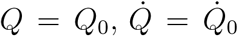 for an initial time t = t0. The stream wise centerline penetration distance and velocity for the jet and puff stages can be given by [100, 101]:
for an initial time t = t0. The stream wise centerline penetration distance and velocity for the jet and puff stages can be given by [100, 101]:
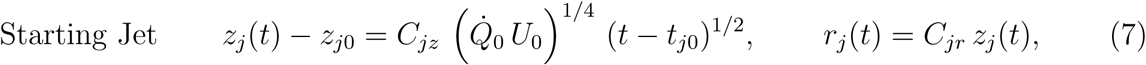

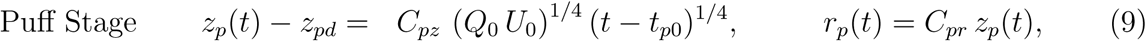
 where the constants Cjz, Cjr, Cpz, Cpr are empirically determined, and zj0 is the z coordinate value of the ejection orifice and zpd is the virtual origins of the puff (see Figure 2), which is an appropriate parameter to separate the starting jet and puff stages though it lies within the starting jet region (see detailed explanation in [100]). For the axial geometry of the jet/puff system under consideration we have
where the constants Cjz, Cjr, Cpz, Cpr are empirically determined, and zj0 is the z coordinate value of the ejection orifice and zpd is the virtual origins of the puff (see Figure 2), which is an appropriate parameter to separate the starting jet and puff stages though it lies within the starting jet region (see detailed explanation in [100]). For the axial geometry of the jet/puff system under consideration we have  and
and 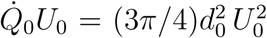 Following [100, 101], we will choose the following numerical values for the constants in (7)–(10): Cjz = 2.8, Cjr = 0.15, zj0 = d0/(2Cjr) and Cpz = 2.6, Cjr = 0.17, while the time and position of the puff virtual origin follows from zpd = zj(texh) − 8.5d0, with tpd determined numerically from (7) by the condition zj(tpd) = zpd [100, 101]. Many vapers exhale at a downward angle typically γ ∼ 30 degrees, thus reducing the horizontal penetration of the starting jet given by (7) roughly as zj cos γ.
Following [100, 101], we will choose the following numerical values for the constants in (7)–(10): Cjz = 2.8, Cjr = 0.15, zj0 = d0/(2Cjr) and Cpz = 2.6, Cjr = 0.17, while the time and position of the puff virtual origin follows from zpd = zj(texh) − 8.5d0, with tpd determined numerically from (7) by the condition zj(tpd) = zpd [100, 101]. Many vapers exhale at a downward angle typically γ ∼ 30 degrees, thus reducing the horizontal penetration of the starting jet given by (7) roughly as zj cos γ.
We display in figures 3a and 3b the horizontal displacement or penetration distance and centerline velocity for various initial velocities U0 that characterize several puffing intensities. Notice that the maximal penetration is basically afforded by the momentum trust of the starting jet, with the puff slowly evolving at small almost constant speed. Horizontal penetration varies from 0.5 meters for Mouth Puffing (U0 = 0.5 m/s) through the range between 0.6 and 1.6 meters the MTL regime (U0 = 1 − 2.5 m/s) and beyond 2 meters for the higher intensity DTL regime (U0 = 3 − 4 m/s). Centerline velocity drops to about 0.2 m/s at different times and distances when fluid injection stops in all cases.
Given its short time duration and close distance scope of the momentum trusted staring jet, the analytic model (7)–(8) remains a reasonably good approximation to infer the necessary distance to minimize the risk of direct exposure of bystanders to respiratory droplets. As the jet evolves while fluid is injected there is increasing entrainment from the surrounding air at velocity Ue ∝ Ur, with entrained air reaching about 40% of the jet mass at the end of injection in the transition towards the puff (around its virtual origin) [98, 99]. Since there are airflow currents of ∼ 10 cm/s (and up to 25 cm/s) even in still air in home environments with natural ventilation [105, 106], at this stage the puff formation can be easily destabilized by vortex motion generated through turbulent mixing from the large velocity fluctuations produced by the entrainment [107, 108].
Turbulence and thermal buoyancy become important factors when there is human motion or walking [109], or in micro-environments with mechanical ventilation (mixed or displaced) [110, 111, 112], resulting in a faster disruption and dispersion of the slow moving puff, carrying the submicron ECA and respiratory droplets along the air flow. In general, submicron droplets exhaled at the velocities under consideration can remain buoyant for several hours, with mixing ventilation tending to uniformly spread them, whereas directed ventilation tends to stratify them along different temperature layers. In all cases there is a risk of indirect contagion by exposure to these droplets. The detailed description of droplet dispersion after the puff is disrupted is a complicated process that requires computational techniques that are beyond the scope of this paper (see comprehensive analysis in [108]).
9. A simplified risk model
We have evaluated the distance spread in which exhaled ECA can produce direct contagion by horizontally spreading overwhelmingly submicron respiratory droplets, which once reaching the turbulent puff regime remain buoyant for hours, possibly producing indirect contagion as they are carried by indoor air currents several meters (see comprehensive analysis in [108]). So far we have considered generic respiratory droplets without reference to a specific pathogen/disease and have not evaluated infection risks of exposed susceptible individuals. We undertake now this evaluation, referring specifically to the available information on the parameters of the SARS-CoV-2 virus, assuming as well that submicron respiratory droplets or droplet nuclei potentially carrying this virus have been dispersed uniformly throughout a given indoor micro-environment.
The most important feature that fully characterizes exposure risks from vaping expirations is the significant shortening of exposure time because of their intermittent and episodic nature: an infectious vaper (symptomatic or not) would emit respiratory droplets only while vaping (120-200 daily exhalations [16, 62]), whereas the same vaper will emit respiratory droplets continuously just by normal rest breathing (17,000–29,000 daily exhalations for 12-20 breaths per minute for healthy adults).
9.1. Infective quanta
To evaluate indirect exposure risks from vaping we simplify and adapt the analytic risk model of Buonanno, Morawska and Stabile (hereafter BMS) [15] that has examined the potential SARS-CoV-2 virus transmission in various indoor micro-environments (see also their previous paper [14]). BMS develop this model by means of Montecarlo simulations in which variability of droplet emission rates and exposure parameters is described by suitable probability distributions. Our approach is to assume median values for these variables (50 percentiles) of these distributions, similar to their approach in their previous paper [14]. This is justified because our aim is to evaluate the risks from indoor COVID-19 transmission from vaping, speaking and coughing (all episodic or intermittent expirations) in comparison with what can be denoted as a “control case” scenario of risks in a space were the infectious vaper is only rest breathing (a continuous expiration). We are not aiming at providing a full comprehensive risk analysis for each respiratory activity separately under more realistic conditions (something that would justify a full separate study in itself).
BSM consider the notion of an infective “quantum”: the dose of airborne respiratory droplet nuclei necessary to infect 63% of exposed susceptible individuals. They introduce the “quantum emission rate” ERq (emitted quanta per hour) for various respiratory expirations
 where cv is the viral load (RNA copies/mL) in the sputum of a SARS-CoV-2 infected person (symptomatic or not), cRNA is the number of RNA copies per PFU (plaque forming unit) needed to generate infection and cPFU is quanta-to-PFU conversion parameter, fbr is the number of breaths per hour and VT the tidal exhaled volume, Cd is the droplet volume concentration (in mL/m3, hence Cd VT is the total volume of exhaled droplets in mL). BMS define the product “IR = VT × fbr” as an “inhalation rate”, but it is really an exhalation rate expressible in units m3/h.
where cv is the viral load (RNA copies/mL) in the sputum of a SARS-CoV-2 infected person (symptomatic or not), cRNA is the number of RNA copies per PFU (plaque forming unit) needed to generate infection and cPFU is quanta-to-PFU conversion parameter, fbr is the number of breaths per hour and VT the tidal exhaled volume, Cd is the droplet volume concentration (in mL/m3, hence Cd VT is the total volume of exhaled droplets in mL). BMS define the product “IR = VT × fbr” as an “inhalation rate”, but it is really an exhalation rate expressible in units m3/h.
For the infection parameters BMS consider values that have emerged from recent data: cv = 107 RNA copies/mL (average in the range 103 − 1011), cRNA = 1.3 × 102 RNA copies/PFU and cPFU = 2.1 × 102 PFU/quanta. For the droplet volume concentration they take as reference an experimental value that incorporated dehydration effects in droplets associated with loud speech [113], then using experimental data from Morawska et al [84] to scale this reference to other respiratory expirations, leading to the following values (in mL/m3)
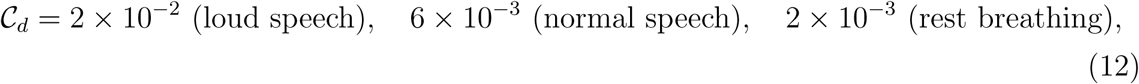
In order to fit vaping expirations into these values we need to make some assumptions on the involved parameters, besides considering the effects on exposure from the time duration of expiratory activities. In particular, we need to evaluate their mean quanta emission rate only in the times when they occur and compare with the rates of normal rest breathing (which takes place all the time). To simplify matters, we assume that cv, cI and f(br) are largely unaffected by the timing of these expiratory activities. We have then
Low intensity MTL Vaping
A vaper breathes N(tot) times in (say) one hour and of these breaths N(vp) coincide with vaping expirations (puffs), the expression for ERq in (11) must be modified as
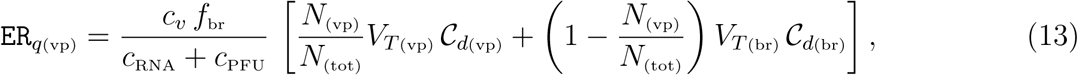 where N(vp) N(tot) are the number of vaping puffs and total number of breaths per hour, VT(br)VT (vp) and Cd (vp), Ci(br) are the tidal volumes and droplet volume concentration for vaping and rest breathing. For low intensity MTL vaping we assume a tidal volume of VT = 750 cm3 supported by inference from data discussed in previous sections, while for droplet volume concentration we assume Cd = 3 × 10−3 mL/m3, a plausible value denoting emissions slightly above rest breathing but below normal speech in (12), fitting the ‘whispered counting’ data of [84]. For the number of breaths we can take the average values of 160 daily puffs in a 16 hour journey [16, 62] and breathing frequency of f(br) = 16/min (in the range 12-20), so that N(tot) = 960 breaths/h and N(vp) = 10 breaths/h.
where N(vp) N(tot) are the number of vaping puffs and total number of breaths per hour, VT(br)VT (vp) and Cd (vp), Ci(br) are the tidal volumes and droplet volume concentration for vaping and rest breathing. For low intensity MTL vaping we assume a tidal volume of VT = 750 cm3 supported by inference from data discussed in previous sections, while for droplet volume concentration we assume Cd = 3 × 10−3 mL/m3, a plausible value denoting emissions slightly above rest breathing but below normal speech in (12), fitting the ‘whispered counting’ data of [84]. For the number of breaths we can take the average values of 160 daily puffs in a 16 hour journey [16, 62] and breathing frequency of f(br) = 16/min (in the range 12-20), so that N(tot) = 960 breaths/h and N(vp) = 10 breaths/h.
High intensity DTL vaping
We assume VT = 2000 cm3 as an average tidal volume. However, there is ambiguity in inferring a value for droplet volume concentration because of insufficient data on how much the larger tidal volume and deeper inhalation of DTL vaping can modify respiratory droplet numbers and diameters. As mentioned in section 3, higher powered devices associated with DTL vaping tend to increase ECA droplet sizes and diameters [26, 22] but it is not certain if this applies to respiratory droplets. However, as mentioned in section 7, speech involves droplet generating mechanisms that are distinct from those of breathing [91, 84, 82], resulting in higher rate of droplet emission even with a tidal volume only slightly larger than the breathing rest value of 400 − 600 cm3 [114, 115]. Thus, we have two plausible options to account for a higher total volume of exhaled droplets 𝒱d = VT 𝒞d: it may follow simply from a larger VT with the same value 𝒞d = 3 × 10−3 mL/m3 of low intensity vaping, or we might assume the larger value of Cd for normal speech in (12). Instead of choosing one option, we will keep the continuous range of Cd = 3 − 6 × 10−3 mL/m3. Regarding the number of breaths we can assume the same values as low intensity vaping: N(tot) = 960 breaths/h and N(vp) = 10 breaths/h.
Normal speech
The equation for ERq in (11) needs to be modified in a similar way as (13), replacing the droplet volume concentration Cd with the value for normal speech in (12) and we take as tidal volume the value VT = 600 cm3, roughly 10% larger than the average rest value [114, 115]. To incorporate the timing we replace N(vp) with a number count of breaths coinciding with a given percentage of an hour interval spent on continuously speaking at home or in a restaurant. For 5, 10, 20, 30, 40% of the hour (960 total breaths) we have N(sp) = 48, 96, 192, 288, 384 breaths/h.
Coughing
The emission data from coughing in [84] is comparable to that of ‘unmodulated vocalization’ (repeating the vowel “aahh”). Hence, we can use (13) with the value for droplet concentration volume of loud speaking in (12) as a proxy for coughing, while for coughing tidal volume we have VT = 1400 cm3 [44]. Assuming a cough every 2 and 3 minutes, N(vp) is replaced by N(cf) = 20, 30.
Considering the plausible assumptions stated above, we display in figure 4 the logarithmic plots of quanta emission rate ERq from an infectious individual as a function of viral load cv, for rest breathing, low and high intensity vaping, speaking for 10%, 20%, 30% and 100% of the time, as well as coughing every 2 and 3 minutes. The numerical values of ERq in quanta per hour for cv = 107 RNA copies/mL are 0.3416 (breathing), 0.3562, 0.3727 − 0.4139 (vaping low and high intensity), 0.5063, 0.6610, 0.8158, 0.9705 (speaking 10, 20, 30, 40% of the hour), 1.2637 (coughing 30 times) and 1.890 (speaking 100% of the hour). Notice that for low and high intensity vaping ERq is very close to the control case of rest breathing (almost indistinguishable for low intensity vaping), while even speaking 10% of the hour (6 minutes) yields a larger ERq value than the upper end of high intensity vaping. Also, normal speech for a full hour (not uncommon) produces a higher quanta emission than coughing 30 times
9.2. Exponential dose-response risk model
In order to evaluate a time dependent risk for expiratory activities that incorporates quanta emission rates and indoor environment variables, BSM consider the “dose response exponential model” given in terms of the the density of the quanta n(t) in units quanta/m3 under the assumption that n(0) = 0 (no exposure at initial time t = 0)

 where V is the volume (m3) of the indoor micro-environment, N is the number of exposed susceptible individuals, IR is the inhalation rate (m3/h) of these individuals and IVVR is the infectious virus removal rate, which which BMS take as the sum of three factors: IVVR = AER + κ + λ, where AER is the ventilation air exchange rate, κ is the particle deposition on surfaces and λ is the virus inactivation (all of these quantities given as h−1).
where V is the volume (m3) of the indoor micro-environment, N is the number of exposed susceptible individuals, IR is the inhalation rate (m3/h) of these individuals and IVVR is the infectious virus removal rate, which which BMS take as the sum of three factors: IVVR = AER + κ + λ, where AER is the ventilation air exchange rate, κ is the particle deposition on surfaces and λ is the virus inactivation (all of these quantities given as h−1).
In order to apply (14) we use the value IR = 0.96m3/h taken BMS’s previous paper and justified as a level of physical activity half way between standing and light activity. For the remaining parameters BSM assume the range AER = 0.2 − 0.5/h for natural ventilation and AER = 9.6/h for a restaurant scenario with mixed ventilation.
They compute the deposition rate by dividing typical gravitational settling velocity for supermicron particles (10−4 m/s) by the height of emission (1.5 m), leading to κ = 0.24/h, while for the viral inactivation they take the measured aerosolized SARS-CoV-2 virus mean life of 1.1 hours [116], leading to λ = 0.63/h. We consider the following home and restaurant indoor scenarios:
Home scenario. We assume one infectious vaper and three exposed susceptible family members (N = 3). Total exposure time T = 12 h. Indoor volume 125 m3 (small 50 m2 apartment with roof height of 2.5 m). For natural ventilation: AER = 0.2/h we have IVVR = 1.07/h.
Restaurant, natural ventilation with open door. Thirty costumers (N = 30), total exposure time T = 3 h. Air exchange rate AER = 0.5/h, indoor volume 300 m3 (100 m2 area with roof height of 3 m), results in IVVR = 1.37/h
Same restaurant endowed with mechanical ventilation: AER = 9.6/h (taken from [14]), results in IVVR = 10.47/h
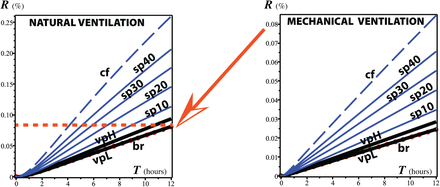
The infection risk R for home and restaurant scenarios is plotted in figures 5 and 6 as a function of time for breathing, low and high intensity vaping, various percentages of time spent speaking and coughing every 2 minutes, considering natural and mechanical ventilation. As expected from the quanta emission rates displayed in figure 4, the exposure time of different expirations is a crucial factor in computing R. Exposure to vaping expiration (vaper doing 10 puffs per hour) poses an infection risk to bystanders that is very close to that from the control case scenario: exclusive normal rest breathing (for low intensity vaping the infection risk is practically indistinguishable). The infection risk from a person vaping is well below that from the same person speaking and coughing: speaking only for 10% of the time (6 minutes per hour) already yields a higher infection risk than high intensity vaping, while speaking 30 − 40% yields up tp 4 times the infection risk, which is roughly the values plotted in figure 7.
The curves display R as a function of exposure time T from (14). The abbreviations br, vpL, vpH, sp10, sp20, sp30, sp40 and cf stand for rest breathing, vaping low intensity, vaping high intensity (upper end option), speaking for 10, 20, 30, 40,% of time and coughing. Notice the dramatic reduction of R achieved by mechanical ventilation (air exchange rate of 3=h). Also: the curves for the risks from vaping (all intensities) are practically indistinguishable from that of the case control scenario of rest breathing (red circles).
The same abbreviations as in figure 5 plus sp100 (speaking 100% of the time, a possible outcome when spending 3 hours in a restaurant). As in figure 5, mechanical ventilation (air exchange rate 9:6=h) achieves a dramatic reduction of R and the curves for the risks from vaping are practically indistinguishable from the curve of the control case scenario of rest breating (red circles).
The percentage values with respect to the control case are: low intensity vaping 1.3% (vpL), high intensity vaping 5.2 − 17.7% (vpH), speaking 44% (sp10), 88% (sp20), 132% (sp30), 176% (sp40) for 10%, 20%, 30%, 40% of time, coughing 259% 30 times per hour (cf). These values were obtained from (ε − 1) × 100 for ε defined for these expiratory activities by (16) − (18).
A good inference of the risk from intermittent and episodic expiratory activities (vaping, speaking, coughing) relative to the control case scenario of exclusive rest breathing (a continuous expiration) is furnished by the ratio R(A)/R(br), where A = vp, sp, cf. Plotting this ratio from (14)–(15) for every expiratory activity yields near constant curves around the values of the quotients ERq(A)/ERq(br). This is not surprising since ERq is the only variable in R that characterizes the infectious person (the other variables characterize the indoor micro-environment and the exposed susceptible persons). Hence, given the same indoor micro-environment and same number of susceptible individuals, we consider risks relative to the control case scenario of rest breathing in terms of the ratio of quanta emission. Using (13) we have
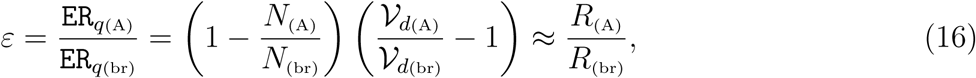 where 𝒱d(A) = VT (A) 𝒱d(A) is the total exhaled droplet volume (in mL) for each expiratory activity. Since N(br) = N(tot), then for a heavy breathing activity in intense aerobic exercise ε might grow only because of the much larger tidal volume. However, for a truly intermittent expiration like vaping we have N(vp)/N(br) ≪ 1 and thus ε ≈ 1 holds even if we have 𝒱d(A)/𝒱d(br) ≫ 1 (large exhaled amount of droplets as with the large tidal volumes in extremely intense vaping). For the values of tidal volume and droplet volume concentration we have used, we have the following relative risks
where 𝒱d(A) = VT (A) 𝒱d(A) is the total exhaled droplet volume (in mL) for each expiratory activity. Since N(br) = N(tot), then for a heavy breathing activity in intense aerobic exercise ε might grow only because of the much larger tidal volume. However, for a truly intermittent expiration like vaping we have N(vp)/N(br) ≪ 1 and thus ε ≈ 1 holds even if we have 𝒱d(A)/𝒱d(br) ≫ 1 (large exhaled amount of droplets as with the large tidal volumes in extremely intense vaping). For the values of tidal volume and droplet volume concentration we have used, we have the following relative risks
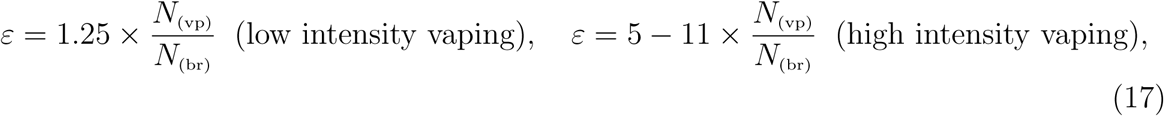
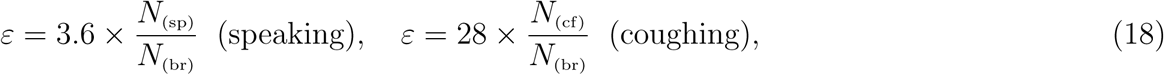 which provides an intuitive indication of the added exposure risks relative to the control case from the different expiratory activities.
which provides an intuitive indication of the added exposure risks relative to the control case from the different expiratory activities.
We display in figure 7 the numerical values of ε, as an added risk with respect to the control case for various expiratory activities with respect to the continuous presence of risk from rest breathing and under the assumptions of we have used. These numbers clearly reflect the effects of the intermittence or duration time of each activity. Under normal vaping conditions (10-15 puffs per hour) the added risk of low intensity vaping respect to the control scenario of exclusive rest breathing is of the order of ∼ 1% (since ε−1 ∼ 10−2). For high intensity vaping it is ∼ 5−17%, given the ambiguity in the range of Vd = VT Cd, still it is of the order of ε − 1 ∼ 5 × 10−2 − 10−1, also a low added risk since the low value of N(vp)/N(br) compensates for the large exhaled tidal volume. Notice that the added risk respect to the control case grows to ∼ 40% just for talking for 10% of the time and easily reaches 90% if talking 40% of the time. Coughing is also intermittent, possibly even more intermittent than vaping, but its large amount of exhaled droplets (large factor of 28 in (18)) can offset this effect. For speaking ε can be large even if normal speech involves a tidal volume close to rest breathing, but it also involves a much larger amount of time (larger number of breaths in typical conversation).
10. Chemical interactions
As mentioned in the Introduction, respiratory droplets potentially carrying the SARS-CoV-2 virus that are exhaled by vapers are not really “airborne” but “ECA–borne”, i.e. they are carried by a completely different chemical environment relative to air diluted plain exhaled breath condensates. It is thus important to discuss the potential effect on the pathogens by known mechanisms of disinfection of glycols such as propylene glycol (PG) and Glycerol or Vegetable Glycerine (VG), which are the main co-solvents used in the process of generation of ECA (and are also the main ingredients in the nicotine containing e-liquids).
Both PG and VG are organic compounds of the family of polyfunctional alcohols, commonly used as drug solubilizers in topical, oral, inhaled, nasal, optical and intravenous medications, as well as water-miscible co-solvents that provide both antimicrobial properties and an increase of the overall stability of many liquid pharmaceutical forms [117, 118, 119, 120]. Since both PG and VG are known hygroscopic compounds, they have been used to induce or sustain desiccation in gases [121], and conversely in liquid formulations to preserve hydration in several applications as humectants [122, 123].
The numerous applications of PG include
Antiseptic: provides antimicrobial activity similar to that of ethanol [118].
As an active ingredient it has been used in air sanitization [119], product preservation [120]
Hard surface disinfection against bacteria, fungi and viruses, while as a food ingredient PG has been used as co-solvent, humectant, rheological modifier [122].
Preservative demonstrating complete bactericidal effects at aqueous concentrations of 25%.
Regarding VG (see [123]), it is also known for its antibacterial [124] and antiviral properties [125] and is used in several pharmaceutical, cosmetic and food applications due to its relative safety, sweet taste, unique humectant properties (more effective than PG because of its larger viscosity [123])
The fundamental mechanisms governing antimicrobial and viral inactivation of VG and PG are still not fully understood, based on the increased efficacy in the presence of water and the dependence of the relative humidity in gases, and water activity in solids and liquids, it is generally believed that these agents can induce microbial membrane damage by dehydration, osmotic effects, phospholipidic membrane and enveloped capsid disarrays caused by hydrophobic-hydrophilic surface alterations, coagulation and denaturation of membrane proteins [126, 127].
The aerial disinfection can be initially attributed to the reduction of water and desiccant activity that VG and PG and other glycols have in aqueous solutions and water-containing vapor systems [128], glycols after condensation can nucleate by adsorption around aqueous bio droplets driven by the electrostatic attraction that they have towards the water and proteins present in these particles, the intensity of the H-OH hydrogen bonding that both PG, VG and other glycols manifest with water in heterogeneous water polyphase systems also facilitate the reduction of water activity, which can subsequently reduce the viability of these microorganisms suspended as aerosols [129].
The bactericidal effect of glycols in vapours has been studied since 1928 [128, 127, 130, 131]. During the 1930’s and 1940’s Puck and Robertson studied the bactericidal and virucidal effects of glycols, particularly PG, acting on several vapor-water systems with
suspended microorganisms. As explained by Puck [127], once the glycols are embedded in the liquid phase of the bioaerosol droplet, a water/glycol equilibrium is reached, with glycol and water diffusion taking place through the biological membrane, thus inducing membrane alterations and swelling on the viable particles and terminating with inducing microbial osmolysis. Relative humidity and temperature affects the microbicidal effect of glycols, with the most favorable conditions for the biocidal action of PG in its vapor phase given by a temperature below 26° C and relative humidity between 45% and 70% (see comprehensive explanation also in [128, 130]).
Puck and coworkers also found that air diluted PG vapor in concentrations of 250 − 500 mg/m3 induced an immediate and complete sterilization in an environment in which Pneumococci, Streptococci, Staphylococci, H. Influenzae, and other microorganisms were suspended. Concentrations of 210 mg/m3 were sufficient to fully disinfect air in a chamber with suspended Staphylococcus Albus after 10 minutes [128]. Concentrations as low as 50 mg/m3 were effective against Pneumococci. (20). In another study the vaporization of PG was implemented in hospital rooms as preventative mechanism against Streptococcus Haemolyticus, under these more diverse environmental conditions regarding temperature and humidity, concentrations over 100 mg/m3 sustained its bactericidal effect [131].
It is difficult to relate these highly controlled and idealized experiments to the erratic and highly variable conditions in vaping. First, in these experiments pure PG (as aerosol or as vapor) was supplied continuously and spread evenly under carefully controlled conditions, whereas particulate and gas phase concentrations of ECA rapidly vary with time and position. The gas phase of ECA is a mixture of PG and other compounds (VG, nicotine, with residual concentrations of mostly aldehydes) and is supplied into the surrounding air (when inhaled or exhaled) intermittently during puffs and spreads unevenly. Second, bactericidal effects in these experiments were registered with PG concentrations of: 50 − 500 mg/m3 which are 2-3 orders of magnitude higher than maximal gaseous PG concentrations of exhaled ECA registered in experiments involving several users vaping in relatively small chambers during hours: 0.3−0.4 mg/m3 [132, 133]. Since about 92% of inhaled PG is retained [34], PG concentrations inside the respiratory tracts could approach the lower end of concentrations in the experiments. However, the disinfectant effect is unlikely to occur, as the transit and absorption time of ECA in the respiratory system is too short (around 5-6 seconds) and this effect is much less efficient in the prevailing relative humidity close to 100%.
As we have argued throughout this article, exhaled ECA (as an expiratory activity) should spread respiratory droplets in the environment. However, it is very unlikely that its chemical medium could inhibit COVID-19 contagion by disabling or destroying the SARS-CoV-2 virus. Conversely, it is equally unlikely that this chemical medium could (somehow) enhance the probability of contagion in comparison with “normal” airborne transmission. Nevertheless, the chemical interaction between the SARS-CoV-2 virus and PG and/or other glycols and compounds of ECA needs to be probed and tested in well designed experiments, even outside the context of vaping.
11. Final summary, study limitations and conclusion
We have provided in this paper a comprehensive study of the plausibility, scope and risk for pathogen (including SARS-CoV-2 virus) contagion associated with respiratory droplets that would be carried by ECA (e-cigarette aerosol) exhaled by vapers.
11.1. Final summary
In what follows we provide a summary of the main results we have found. To examine the plausibility of respiratory droplets carried by exhaled ECA we took first into consideration basic specific characteristics of vaping, such as
The wide diversity of vaping styles or puffing topographies
To deal with this complexity we provide (section 2) a rough simplified classification in two main categories: the majority (80-90%) usage low intensity ‘mouth-to-lung’ (MTL) and the minority usage (80-90%) high intensity ‘direct to lung’ vaping.
Respiratory vs ECA droplets
Respiratory droplets emitted by vapers would be accompanying a vastly larger number (about ∼ 108 − 109) of rapidly evaporating droplets of the particulate phase of exhaled ECA, made of propylene glycol (PG), glycerol or vegetable glycerine (VG), nicotine and water. The respiratory and ECA droplets would be suspended in a carrier fluid distinct from that of respiratory droplets without vaping: the gas phase of exhaled ECA strongly diluted in air (since retention of inhaled ECA by the respiratory system is about 90%).
ECA as a visual tracer of respiratory flows
Once exhaled ECA evolves as a single-phased fluid flow (section 4) in which the submicron (respiratory or ECA) droplets exert negligible influence on the dynamics of the carrier fluid (the ECA gas phase diluted in air). As a practical consequence, ECA droplets can be regarded as visible tracers of the exhaled air flow as other tracing gases and aerosols [42, 43].
Given the lack of experimental data, we need to infer the size and rate of emission of respiratory droplets that would be carried by exhaled ECA by looking at available evidence on expiratory activities that can serve as proxies for vaping. We proceed along the following steps
Smoking is a useful proxy to estimate the breathing mechanics of vaping
This assumption is justified since most vapers are relatively recent ex-smokers and many are still current smokers, mostly following the MTL vaping style that resembles the puffing topography of most cigarette smokers. From the available evidence (section 5) we estimate that MTL vaping should involve an exhaled tidal volume (a key parameter) comparable to that of smoking, which is about 30-40% larger than that for normal rest breathing (roughly 700-900 vs 400-600 cm3). For DTL vaping we estimate an even higher exhaled tidal volume (1000 − 3000 cm3), given the larger volume of inhaled puffing volume and aerosol mass that it involves.
Vaping involves oral inhalation by suction through a mouthpiece
In section 6 we examined by means of the available literature the interplay between these variables not present in rest breathing. Oral inhalation with a mouthpiece increases tidal volume and inhalation/exhalation times, but these effects practically vanish when nasal breathing is not occluded, as is the case in vaping (and smoking). Hence, the detected increase of tidal volume in smoking (which we assume valid for vaping) is most likely the consequence of the increase of lung volume required for suction. This is consistent with the empiric fact that smoking occupies a higher percentage of vital capacity (roughly 20% as opposed to 10-15% in rest breathing).
Mouth breathing is a useful proxy to infer respiratory droplet diameters and emission rates by vaping
This follows from the fact that its estimated exhalation velocities (30 − 250 and 125 − 400 cm/s for MTL and DTL styles) are comparable to measured velocities for mouth breathing, which are below velocities for speaking, coughing and sneezing reported in the literature. We examine in section 7 available data on respiratory droplets from breathing experiments at different levels of inspiration, with subjects breathing in all cases through mouthpieces and noseclips (whose effects we examined in section 6). We infer from this literature (and considering arguments from sections 5 and 6) the following characteristics of respiratory droplets associated with vaping
MTL vaping should emit on average 2–230 droplets per puff overwhelmingly in the submicron range, a comparable amount of droplet numbers and sizes as the respiratory experiments for tidal volumes close to rest breathing.
DTL vaping should emit on average several hundreds and up to 1000 droplets also in the submicron range but with higher mean diameters. Here the comparative reference is respiratory experiments at more intense level of inspiration.
While the inferred droplet numbers in the upper end of high intensity DTL vaping can be comparable with low end numbers for vocalizing, the latter involves modes with larger mean diameters because of distinct droplet generation processes.
Having inferred the exhaled tidal volume and numbers and diameters of respiratory droplets that should be carried by exhaled ECA, we proceed to estimate:
Distance for direct exposure
To estimate how far can exhaled ECA carry respiratory droplets, we model (section 8) ECA flow as a puff with a starting turbulent jet with finite fluid injection (finite exhalation time). We find that droplets (ECA and respiratory) should be transported horizontally 0.5-2.0 meters for MTL vaping and over 2 meters for DTL vaping. Once the injection stops the jet evolves into an unstable puff that becomes rapidly disrupted by entrained air and turbulent mixing, with the submicron droplets (ECA and respiratory) transported by the jet subsequently dispersing being carried by air currents, thus representing a potential risk of indirect contagion.
Risk of indirect contagion
To asses this risk we consider in section 9 a simplified version of the dose-response exponential risk model presented by Buonanno, Morawska and Stabile [15] (BMS). Our aim is to estimate the relative risk for indoor vaping, an intermittent expiratory activity, in direct comparison with rest breathing, which is an unavoidable continuous expiratory activity that can be considered as a “control case” scenario. The same risk comparison with respect to this “control case” can be estimated for speaking and coughing, which are also episodic and intermittent activities. Assuming that the submicron respiratory droplets have been spread uniformly and considering recent data used by BMS on SARS-CoV-2 viral load and other infection parameters, as well as their data on droplet size and emission rates, we evaluate these relative risks for a home and restaurant scenarios (12 and 3 hours exposure) with natural and mechanical ventilation. The resulting values of added risks with respect to the control case are (see also figure 7):
1% for low the dominant intensity MTL vaping (160 daily puffs)
5-17% for the minority high intensity DTL vaping (160 daily puffs)
44% for continuous speaking 10% of time (6 minutes every hour), up to 90% for speaking 40% of time (20 minutes)
over 260% for coughing 30 times per hour.
ECA as a disinfectant
PG and VG, the main chemical compounds of ECA, are glycols with laboratory tested aerial disinfectant effects on various pathogens (mostly bacteria). After reviewing the literature on this issue, specially on PG (section 10), we conclude that the highly idealized conditions and PG concentrations under which these disinfectant experiments were conducted could hardly be applicable to the erratic and intermittent conditions of realistic vaping. Nevertheless, it should be worthwhile and useful to test experimentally the disinfectant properties of PG on the SARS-CoV-2 virus.
11.2. Limitations
It is important to openly recognize the main limitation of this study: the lack of experimental and observational data on respiratory droplets carried by exhaled ECA. It is quite plausible that emission of these droplets should occur, as exhaled ECA is an expiratory activity, but without empiric data any quantitative assessment of its nature and scope must necessarily be inferred or estimated indirectly, either through theoretical speculation from the physical and chemical properties of ECA, or through extrapolation from available data on other expiratory activities that can serve as reasonable proxies for vaping. The need to provide the best possible and self consistent inference on this missing data explains and justifies the length of the present study: data availability would render several sections (for example sections 5, 6 and 7) redundant or drastically shortened and kept only for comparative reference.
The classification of puffing topographies in two separate mutually exclusive categories (MTL and DTL) that we presented in section 2 roughly conveys the two main vaping styles, but e-cigarettes are a rapidly changing technology and thus this simplified approach cannot capture the full range and scope of individual vaping habits. While the simple dynamical modelling of exhaled ECA as a starting jet followed by an unstable puff (section 8) is sufficient to estimate direct exposure distances, we recognize its limitations: it is strictly valid for a jet/puff system emitted by a static vaper in still air. Evidently, to estimate the fluid flows that determine indirect exposure requires a more realistic description using computational methods of fluid mechanics to incorporate effects of turbulence and thermal bouyancy, as well as air currents from ventilation or motion. Rather, we examine indirect exposure through a risk model not involving fluid dynamics. It is important to mention that this simplification of the dynamics is harder to justify for expiratory activities like coughing or sneezing, as the latter involve larger ejection velocities and a much wider spectrum of droplet diameters that includes significant number of large supermicron droplets (significant numbers of diameters 5 − 10 µm and even > 100 µm) whose effect on the dynamics of the carrier fluid cannot be neglected (these are strictly speaking multiphasic flows [40, 78, 102]).
The simplified BMS risk model that we presented in section 9 fulfills our aim of providing a rough estimation of relative risks from indirect exposure to intermittent vaping expirations with respect to the control case of continuous rest breathing. However, we do recognize its limitations: the risks are evaluated for a single vaper in highly idealized micro-environments, assuming constant infection parameters and inhalation rates (which BMS also assume), ignoring as well the probabilistic distribution of the quanta emission rates and other parameters (which the model of BMS does incorporate). A more elaborate and complete approach should include a more robust methodology to quantify exposure risks to intermittent and sporadic sources, as for example in [43, 134]. This task is left for a future analysis.
11.3. Conclusion and policy recommendations
Since ECA can be used for respiratory airflow visualization (section 4) and it can also transport respiratory droplets potentially carrying pathogens (including SARS-CoV-2), this fact has an important psychological dimension that is absent in other expiratory activities that also transport such droplets but whose respiratory flow cannot be visualized (speaking, singing, coughing, sneezing). The fact that bystanders are able to visualize respiratory flow through ECA allows them to position themselves at appropriate distances to avoid direct exposure (1-2 meters), similar to recommended social separation distance. This visualization makes it abundantly clear that direct exposure risk applies to distances only in the direction of the exhaled jet, with individuals positioned in other directions only risking indirect exposure. Nevertheless, it is prudent to maintain 2 meters of separation from anyone vaping when not wearing a face mask.
We have shown in section 9 that vaping will add only a minuscule additional risk to those risks already existing from continuous breathing or talking in indoor or socially shared spaces without universal wearing of face masks, which offer fairly effective protection against pathogen contamination by infected persons, but also provide reasonably good protection for bystanders exposed to emissions from people infected who are not wearing a face mask [135].
Evidently, universal face mask wearing in a given socially shared indoor space implies a drastic reduction of the existing baseline risk for continuous breathing (but more so for talking). It can be argued that in such an indoor environment vaping would increase exposure risks, but vaping would not be the only activity causing an increase of risk: eating and drinking are impossible without fully removing face masks, whereas vaping is possible by momentary adjusting a face mask (not necessarily its full removal). However, if face mask wearing is universal this risk enhancement would be negligible and inconsequential because the same face masks would protect those wearing them from short intermittent emissions by others when eating or drinking (and including those from the vaper).
Breathing emissions due to brief intermittent removal or adjustment of a face mask to drink, eat or vape, or even to take a brief rest from wearing the mask, would imply for bystanders (already protected for wearing face masks) to tolerate a small rate of droplet emission only for a very short time. Since face mask cannot be rigidly maintained 100% of time in shared spaces, this tolerance is necessary for civilized coexistence. In the specific case of vaping it implies a tolerance of mask-free periods that would be of shorter duration than those for eating or dinking: likely no longer than 10 seconds roughly 10-15 times per hour (being free from this exposure for the remaining 600-1400 breaths by the vaper in the same hour). It is true that vaping might introduce risks from face touching, or mask manipulation or sharing or manipulating a device that will be placed in the mouth, but the same risks are present (and are tolerated or addressed by hygiene prevention) when drinking or eating. The same tolerance and courtesy given for these acts can (and should) be extended to vapers, most of whom are trying to stop smoking and stay smoke-free (or at least to smoke less).
The risk for direct and indirect COVID-19 contagion from indoor vaping expirations does exist and must be taken into consideration. However, this risk must be placed in its proper context with respect to the parameters of exposure that characterize vaping and other expiratory activities. Therefore, as far as protection against SARS-CoV-2 virus is concerned, vaping in a home scenario or in social spaces does not require special extra interventions besides those already recommended for the general population: social distance and wearing face masks. Vapers should be advised to be alert to the worries and fears of non-vapers when sharing indoor spaces or dwellings or when close to other citizens, and for safety measures to use low-powered devices for low intensity vaping. Vapers, however, deserve the same sensitivity, courtesy and tolerance as well.
Data Availability
The data that support the findings of this study are available from the corresponding author, RAS, upon reasonable request.
Competing interests
RAS has no competing interests to declare.
EG is currently employed by Myriad Pharmaceuticals, an independent company that manufactures e-liquids and vaping devices in New Zealand. She also provides consultancy work on research and development, regulatory affairs support, and formulation to several independent vaping companies in the Pacific Region. In the past she has worked for several pharmaceutical companies, including GlaxoSmithKline and Genomma Lab. She is also a member of the standards committee of the VTANZ and UKVIA.
RP is full time employee of the University of Catania, Italy. In relation to his work in the area of tobacco control and respiratory diseases, RP has received lecture fees and research funding from Pfizer, GlaxoSmithKline, CV Therapeutics, NeuroSearch A/S, Sandoz, MSD, Boehringer Ingelheim, Novartis, Duska Therapeutics, and Forest Laboratories. He has also served as a consultant for Pfizer, Global Health Alliance for treatment of tobacco dependence, CV Therapeutics, NeuroSearch A/S, Boehringer Ingelheim, Novartis, Duska Therapeutics, Alfa-Wassermann, Forest Laboratories, ECITA (Electronic Cigarette Industry Trade Association, in the UK), Arbi Group Srl., and Health Diplomats. RP is the Founder of the Center of Excellence for the acceleration of Harm Reduction at the University of Catania (CoEHAR), which has received a grant from Foundation for a Smoke Free World to develop and carry out 8 research projects. RP is also currently involved in the following pro bono activities: scientific advisor for LIAF, Lega Italiana Anti Fumo (Italian acronym for Italian Anti Smoking League) and Chair of the European Technical Committee for standardization on Requirements and test methods for emissions of electronic cigarettes (CEN/TC 437; WG4)
Footnotes
E-mail: sussman{at}nucleares.unam.mx, eliana.golberstein{at}myriad.nz, polosa{at}unict.it
↵‡ This 5 μm cut-offs not based on any specific property of aerosol physics, it is a purely conventional value that simplifies into two mutually exclusive modalities the dynamical effects of droplet emission that vary along a continuous spectrum of droplet diameters.
↵§ This paper will address potential COVID-19 contagion through respiratory droplets carried by vaping exhalations, not by environmental tobacco smoke, though smoking can serve as a useful proxy for low intensity ‘mouth to lung’ vaping style. Most of the results we obtain are applicable to “mainstream” smoking exhalations emitted by smokers, not to sidestream emissions from the burning/smouldering tip of cigarettes, cigars and pipes that make the bulk of environmental tobacco smoke.
↵‖ These machine puff time lapses are different from those reported in Table 1. The former correspond only to inhalation times as instruments aim at simulation of a mouth inhalation, the latter are time lapses in human vapers and thus include inhalation and exhalation.
References
- [1].↵
- [2].↵
- [3].↵
- [4].↵
- [5].↵
- [6].↵
- [7].↵
- [8].↵
- [9].↵
- [10].↵
- [11].↵
- [12].↵
- [13].↵
- [14].↵
- [15].↵
- [16].↵
- [17].↵
- [18].↵
- [19].↵
- [20].↵
- [21].↵
- [22].↵
- [23].↵
- [24].↵
- [25].↵
- [26].↵
- [27].↵
- [28].↵
- [29].↵
- [30].↵
- [31].↵
- [32].↵
- [33].↵
- [34].↵
- [35].↵
- [36].↵
- [37].↵
- [38].↵
- [39].↵
- [40].↵
- [41].↵
- [42].↵
- [43].↵
- [44].↵
- [45].↵
- [46].↵
- [47].↵
- [48].↵
- [49].↵
- [50].↵
- [51].↵
- [52].↵
- [53].↵
- [54].↵
- [55].↵
- [56].
- [57].
- [58].
- [59].
- [60].↵
- [61].↵
- [62].↵
- [63].↵
- [64].↵
- [65].↵
- [66].↵
- [67].↵
- [68].↵
- [69].↵
- [70].↵
- [71].↵
- [72].↵
- [73].↵
- [74].↵
- [75].↵
- [76].↵
- [77].↵
- [78].↵
- [79].↵
- [80].↵
- [81].↵
- [82].↵
- [83].↵
- [84].↵
- [85].↵
- [86].↵
- [87].↵
- [88].↵
- [89].↵
- [90].↵
- [91].↵
- [92].↵
- [93].↵
- [94].↵
- [95].↵
- [96].↵
- [97].↵
- [98].↵
- [99].↵
- [100].↵
- [101].↵
- [102].↵
- [103].↵
- [104].↵
- [105].↵
- [106].↵
- [107].↵
- [108].↵
- [109].↵
- [110].↵
- [111].↵
- [112].↵
- [113].↵
- [114].↵
- [115].↵
- [116].↵
- [117].↵
- [118].↵
- [119].↵
- [120].↵
- [121].↵
- [122].↵
- [123].↵
- [124].↵
- [125].↵
- [126].↵
- [127].↵
- [128].↵
- [129].↵
- [130].↵
- [131].↵
- [132].↵
- [133].↵
- [134].↵
- [135].↵